Research works on compressible Euler equations

As one of the most important partial differential equations, Compressible Euler equations are used to describe gas dynamics and have wide applications such as in the aircraft manufacturing. The major difficulty in analyzing the solution of this system is the formation of discontinu- ities, known as shock waves, even when initial data are smooth. Currently, solutions for compressible Euler equations and hyperbolic conservation laws with small total varia- tion in one space dimension (1-D) are fairly well understood. However, well-posedness and behaviors for 1-D solutions with large amplitude (large data) and multi-D solutions, on which my research focuses, are still widely open.

A picture for shock formation of 1-D solution u(t,x).

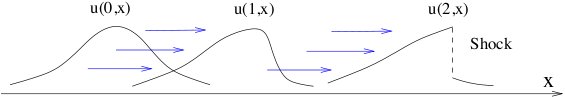
I systematically studied shock formation and structure of large shock-free solutions for compressible Euler equations in 1-D and in a varying duct. Especially, in my very recent papers, by providing a sharp time decay of density lower bound in the order of O(1/t) for classical solutions with large initial data away from vacuum, we generalized Lax's theory (1964) on the shock formation for isentropic Euler equations to include all physical cases with arbitrarily large initial data, and then to full 1-D Euler equations.

A complete resolution on Shock formation for 1-D large solution:

Singularity formation for compressible Euler equations, submitted, (with Ronghua Pan and Shengguo Zhu). pdf
Shock formation in the compressible Euler equations and related systems, J. Hyperbolic Differential Equations, 10 (2013), no. 1, 149-172 (with Robin Young and Qingtian Zhang). pdf
Smooth solutions and singularity formation for the inhomogeneous nonlinear wave equation, J. Differential Equations, 252 (2012), no. 3, 2580-2595, (with Robin Young).
Formation of singularity and smooth wave propagation for the non-isentropic compressible Euler equations, J. Hyperbolic Differential Equations, 8 (2011), no. 4, 671-690.

Sharp lower bound on density in the order of O(1/t):

Optimal time-dependent lower bound on density for classical solutions of 1-D compressible Euler equations, to appear on Indiana University Mathematics Journal. pdf

Structure and long time behavior of shock free solutions:

Shock formation and exact solutions for the compressible Euler equations, Arch. Ration. Mech. Anal., 217:3 (2015), 1265-1293. (with Robin Young). pdf

The existence of large BV (bounded total variation) solutions for the initial value problems to 1-D isentropic (p-system) and full Euler equations is a notoriously difficult and important open problem in the field of fluid dynamics and hyperbolic conservation laws. We obtained several progresses in this field.

By providing a front tracking approximate solution away from vacuum including finite time BV norm blowup, Bressan, Zhang and I showed that currently available approximate solutions will not provide good total variation estimate for even finite time. This work is inspired by an earlier paper with Jenssen providing partial answer to this question. The proof of these results relies on the careful study of wave interactions of p-system.

No BV bounds for approximate solutions to p-system with general pressure law, to appear on J. Hyperbolic Differential Equations, (with Alberto Bressan, Qingtian Zhang and Shengguo Zhu). pdf
Lack of BV Bounds for Approximate Solutions to the p-system with Large Data, J. Differential Equations, 256 (2014), 3067-3085 (with Alberto Bressan and Qingtian Zhang). pdf
No TVD fields for 1-d isentropic gas flow, Comm. Partial Differential Equations, 38 (2013), no. 4, 629-657 (with Helge Kristian Jenssen). pdf

For 1-D full Euler system, we completely resolved pairwise wave interactions.

Pairwise wave interactions in ideal polytropic gases, Arch. Ration. Mech. Anal., 204 (2012), no. 3, 787-836, (with Erik Endres and Helge Kristian Jenssen).

Research works on compressible Navier-Stokes equations

A new field I recently entered is the compressible Navier-Stokes equations. For multi-D solutions on some nonisentropic compressible Navier-Stokes equations, in collaboration with Zhang and Zhu, we proved the existence of unique local strong solutions for initial boundary value problem possibly including vacuum. Moreover, we proved a minimum principle on the temperature, The paper is available at pdf.